Math: Parabolic Art and Geometry
Students will work in pairs to graph Parabolic Curves over 4 quadrants of an x- and y- axis, and then create a series of conjectures about Parabolic Curves based on their measurement of lines, angles, symmetry and patterns. The resulting product will be similar in appearance to string art.
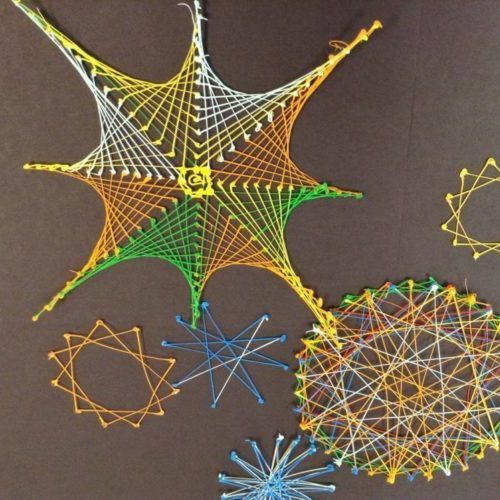
KnowledgeStudents havemeasured angles with a protractor.
measured lines with rulers.
measured angles with a protractor.
measured lines with rulers.
ObjectivesStudents willidentify axes of symmetry.
recognize that straight lines can form a curved profile.
identify axes of symmetry.
recognize that straight lines can form a curved profile.
MaterialsStudents will needruler (1 per pair)
protractor (1 per pair)
pencil (1 per student)
graph paper (1 per pair)
double sided tape (1 per pair)
bamboo skewers (14 per pair)
ruler (1 per pair)
protractor (1 per pair)
pencil (1 per student)
graph paper (1 per pair)
double sided tape (1 per pair)
bamboo skewers (14 per pair)
Lesson PlanInstructions
Step 1Whole group: Challenge the class to create a curve from a series of straight lines using bamboo or any type of wooden skewers.
Step 2After giving students some time to practice, introduce them to the concept of a Parabolic curve.
Step 3Share the goal: Students will create a curve from a series of straight lines using a pencil, ruler and graph paper.
Step 4Model how to do it. Project your computer or tablet screen on the board and display Parabolic Curve Template A. Use a ruler to draw straight lines to connect like numbers on each pair of axes. Show students how to draw a line from number 1 on the x- axis to number 1 on the y- axis. Do the same for each pair of numbers.
Step 5Demonstrate how to use the 3Doodler to connect point to point. When creating a new line, anchor it to the graph paper, then lift the line gently and extend it to the next point anchoring it before ending it. Use a 2-3 color pattern for aesthetics. Allow plastic to harden. Peel off graph paper and adhere to contrasting color construction paper background with double-sided tape or by welding it to the paper with 3Doodled beads of plastic.
Step 6Instruct students to observe patterns, angles and lines of intersection. Record their observations on the board.
Step 7Divide students into pairs. Hand out one copy of Parabolic Curve Template A to each pair. Hand out protractors and rulers. Instruct students to measure lines and angles as they work. Tell students to record their measurements and observations.
Step 8Ask students to come up with a conjecture or rule to explain how to form a Parabolic Curve. Ask: "What do we know to be true?" "Do you need a maximum or minimum number of lines?"Help students understand the following: All lines must be straight. All lines must intersect each other at a 90º angle.
Step 9Rather than share assertions about parabolic curves, lead students to conjectures through an effective line of questioning.
Whole group: Challenge the class to create a curve from a series of straight lines using bamboo or any type of wooden skewers.
After giving students some time to practice, introduce them to the concept of a Parabolic curve.
Share the goal: Students will create a curve from a series of straight lines using a pencil, ruler and graph paper.
Model how to do it. Project your computer or tablet screen on the board and display Parabolic Curve Template A. Use a ruler to draw straight lines to connect like numbers on each pair of axes. Show students how to draw a line from number 1 on the x- axis to number 1 on the y- axis. Do the same for each pair of numbers.
Demonstrate how to use the 3Doodler to connect point to point. When creating a new line, anchor it to the graph paper, then lift the line gently and extend it to the next point anchoring it before ending it. Use a 2-3 color pattern for aesthetics. Allow plastic to harden. Peel off graph paper and adhere to contrasting color construction paper background with double-sided tape or by welding it to the paper with 3Doodled beads of plastic.
Instruct students to observe patterns, angles and lines of intersection. Record their observations on the board.
Divide students into pairs. Hand out one copy of Parabolic Curve Template A to each pair. Hand out protractors and rulers. Instruct students to measure lines and angles as they work. Tell students to record their measurements and observations.
Ask students to come up with a conjecture or rule to explain how to form a Parabolic Curve. Ask: "What do we know to be true?" "Do you need a maximum or minimum number of lines?"Help students understand the following: All lines must be straight. All lines must intersect each other at a 90º angle.
Rather than share assertions about parabolic curves, lead students to conjectures through an effective line of questioning.
Wrap Up
Assessment
Possible Extensions
Resources
Vocabulary
angle - the (rotational) space between two intersecting lines.
collaboration - to work jointly with others or together especially in an intellectual endeavor.
conjecture - a proposition (as in mathematics) before it has been proved or disproved.
geometry - a branch of mathematics that deals with the measurement, properties, and relationships of points, lines, angles, surfaces, and solids; broadly : the study of properties of given elements that remain invariant under specified transformations.
line art - graphic material that consists of lines or (areas of pure black and pure white).
math - the science of numbers and their operations, interrelations, combinations, generalizations, and abstractions and of space configurations and their structure, measurement, transformations, and generalizations.
measurement - the act or process of measuring; a figure, extent, or amount obtained by measuring.
parabolic curves - in mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped. It fits any of several superficially different mathematical descriptions, which can all be proved to define exactly the same curves.
pattern - an artistic, musical, literary, or mechanical design or form.
proofs - in mathematics, a proof is an inferential argument for a mathematical statement.
protractor - an instrument for laying down and measuring angles in drawing and plotting.
rule - a determinate method for performing a mathematical operation and obtaining a certain result.
ruler - a smooth-edged strip (as of wood or metal) that is usually marked off in units (such as inches) and is used as a straightedge or for measuring.
straight lines - an object in geometry that is characterized as a straight, thin, one-dimensional, zero width object that extends on both sides to infinity.
string art - art that is made with strings that is often mathematical in nature, in that it is regularly crafted on an x and y axis.
symmetry - the correspondence in size, form, and arrangement of parts on opposite sides of a plane, line, or point; regularity of form or arrangement in terms of like, reciprocal, or corresponding parts.
Educational Standards
Reason abstractly and quantitatively.
Students will form conjectures about Parabolic Curves based on observations and measurements.
Model with mathematics.
Students will analyze and evaluate by creating a concrete Parabolic Curve with a 3Doodler.
Look for and make use of structures and patterns.
Students will note the structures and patterns of Parabolic Curves through measurement of lines, angles and observations.
Generate and compare multiple possible solutions to a problem based on how well each is likely to meet the criteria and constraints of the problem.
Students will generate and compare multiple 3Doodled Parabolic Curves in order to draft a series of rules and conjectures.
Decompose (break down) a larger problem into smaller sub-problems with teacher guidance or independently.
Students will break down the larger problem of drafting a series of rules and conjectures for Parabolic Curves by 3Doodling, measuring and observing their concrete models.
Use technology to seek feedback that informs and improves their practice and to demonstrate their learning in a variety of ways.
Students will use the 3Doodler to visually demonstrate the the relationship between lines, angles, symmetry and patterns in Parabolic Curves.
Exhibit a tolerance for ambiguity, perseverance and the capacity to work with open-ended problems.
Students will demonstrate willingness and competency within an open-ended task that has more than one possible outcome.
Create original works or responsibly repurpose or remix digital resources into new creations.
Students will use a 3Doodler to design a series of Parabolic Curves.
Use collaborative technologies to work with others, including peers, experts or community members, to examine issues and problems from multiple viewpoints.
Students will use a 3Doodler with a partner to complete a series of Parabolic Curves.





